Voltage, Current, and Resistance - Part 4
Equivalent Resistance (Symbol: Req or req): Series and Parallel Connection
- The term equivalent resistance in electrical circuits denotes the overall resistance that a configuration of resistors offers to a voltage source. The configuration of resistors—whether in series or parallel—impacts the overall resistance.
1. Series Connection:
- In a series connection, resistors are connected end-to-end, meaning the current flows through each resistor sequentially. The total (or equivalent) resistance of resistors in series is simply the sum of their individual resistances.
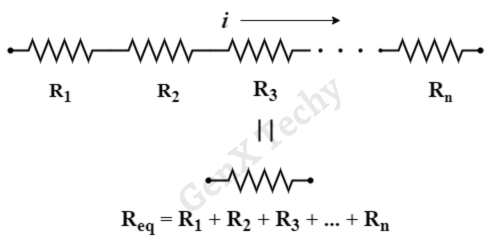
Fig. 1: Equivalent resistance when resistors are connected in series.
The formula for the equivalent resistance, Req in a series connection is:
Req = R1 + R2 + R3 + … + Rn
Example 1: Let’s calculate the equivalent resistance for four resistors connected in series with these values: R1=1Ω, R2=3 Ω, R3=5 Ω, R4=7 Ω
Solution:
The equivalent resistance is:
Req = R1 + R2 + R3 + R4
Substitute the values:
Req = 1Ω + 3Ω + 5Ω + 7Ω = 16Ω
So, the equivalent resistance is 16 Ω
Key Points for Series Connection:
- The current through each resistor is the same.
- The voltage across each resistor is different unless all resistances are equal.
- The total voltage is the sum of the voltages across all resistors.
2. Parallel Connection:
- In a parallel connection, all resistors are connected across the same two points, so the voltage across each resistor is the same, but the current is divided between them.
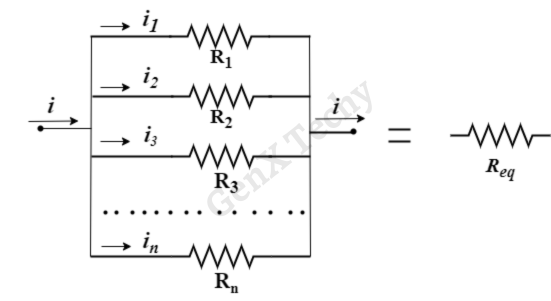
Fig. 2: Equivalent resistance when resistors are connected in parallel.
The formula for the equivalent resistance, Req in a parallel connection is:
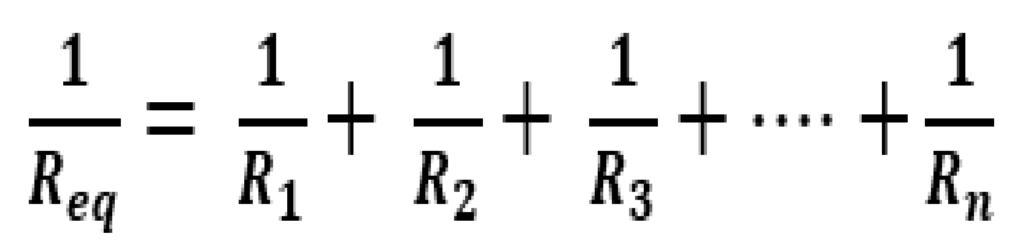
Or, for two resistors:
![]()
Example 2: Let’s calculate the equivalent resistance for four resistors connected in parallel with these values: R1=10 Ω, R2=20 Ω, R3=30 Ω, R4=40 Ω
Solution:
The formula is:
![]()
![]()

![]()
Now, take the reciprocal to find Req:
![]()
So, the equivalent resistance is approximately 4.8 Ω
Key Points for Parallel Connection:
- The voltage across each resistor is the same.
- The current through each resistor is different unless all resistances are equal.
- The equivalent resistance is always smaller than the smallest resistor in the network.
